Introduction
Physics is a subject that makes a person develop the mindset to search for reasons for almost every phenomenon happening around them. From bigger phenomena like black holes, climate change, earthquakes, and natural disasters to very common ones like the flow of water in pipes, the stretching of a rubber band, bouncing of springs, etc. all these involve physics in some or the other way. The following article is about Relationship between Frequency and Energy
The subject of physics is so vast and immensely applicable n real life that it consists of various branches that deal with specific areas and applications. One such branch is Quantum physics, which deals with the behavior of particles or matter. It tells us about the atom’s structure and the energy levels at which particular electrons revolve.
Quantum physics also tells us about the energy, frequency, and wavelength a particle posses according to the very famous French physicist Louis De Broglie particles also depict wave nature.
All these physical quantities are related to each other because of certain reasons and conditions. In this article, we will know more about the relationship between Frequency and energy.
Before diving into the topic let us first know about Frequency and Energy.
Frequency
In fundamental physics and in a general perspective Frequency is the number of occurrences that an event or phenomenon makes while repeating in a particular time period. Frequency has a unit known as Hertz (Hz), it represents one cycle per second.
In quantum physics, when analyzing the wave nature of a particle, we come across the quantities of wavelength, and wave cycles. Frequency is the number of wave cycles that pass a given point in one second.
Frequency is a crucial parameter in various fields of physics, including acoustics, optics, electronics, and quantum mechanics. It plays a significant role in understanding and analyzing wave phenomena, signal processing, communication systems, resonance phenomena, and the behavior of quantum particles.
Formula For Frequency
As stated above Frequency is a parameter that defines the number of cycles an event or phenomenon makes with respect to a given time period, hence if we denote Frequency as f and time period as T then the frequency is equal to:
f = 1/ T
Frequency in this formula has Hertz as its unit and the Time period is measured in seconds. The formula also provides us a conclusion that frequency and time period are inversely proportional to each other.
When a particle possesses a higher frequency it means that, in order to complete one cycle the particle takes lesser time, while a particle having low frequency takes more time for the completion of one cycle.
There exists a relationship that helps [s us to convert or calculate the Frequency of a particle with the help of a given angular frequency. The formula is
f = ω / 2π
Where ω is the angular frequency.
Energy
The measurement of work done or change caused by a body or system is known as Energy. Its unit is Joule (J). Energy is of various types depending upon the conditions and way it is formed or generated.
Forms of energy are:
- Kinetic Energy – Whenever a body is in motion it performs and executes some work, this work is done by the energy present inside the body, hence the energy present in a moving body is termed Kinetic energy.
- Potential Energy – Potential energy is referred to as the Energy stored in an object with respect to its position, when present in a force field.
Gravitational potential energy, elastic potential energy, and Electric potential energy are types of potential energies a body possesses when present in various force fields.
- Thermal Energy – this energy is formed by the internal collisions of particles in a body. As we know particles of an are always in a random motion, due to which they consist of kinetic energy, the average of all kinetic energies is termed as thermal energy. It is also responsible for the temperature of the system.
Formula for energy
As we saw in the above section there are numerous forms of energy that a body may acquire, and physicists after conducting several researches and experiments have found mathematical expressions for the calculation of the appropriate value of energy. Here are some of the expressions or formulae –
- Kinetic energy – K.E. = ½ mv2
where m is mass and v is the velocity of the object.
- Potential energy – P.E. = m*g*h
Where m is mass, g is the acceleration due to gravity, and h is the height of the object or position of the object from a respective point.
- Mechanical Energy – it is the addition of both Kinetic as well as Potential energy.
- Energy = K.E. + P.E.
- Energy = ½ mv2 + m*g*h
Understanding the correlation between Frequency and Energy
The physical quantity of frequency and energy can both be accommodated in a body during motion or work. When the theory of dual nature of particles was formed it was found that the frequency and energy of the particle are related to each other.
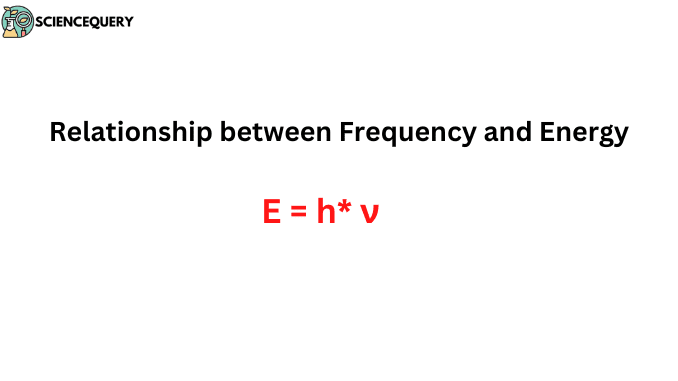
Due to further research and experiments, Planck’s equation gave the mathematical expression for this relation –
E = h* ν
Where E is the energy of the particle or photon, h is Planck’s constant, and ν is the frequency of the electromagnetic wave, as this equation was made taking electromagnetic waves into account
This equation helps us to analyze the fact that those waves which have higher frequency acquire more energy, higher frequency means the particle or wave performs a single cycle in very less time, or for a given time period waves perform more cycles.
On the other hand, when a wave or particle has a lower frequency it results in the acquisition of less energy in the particular or corresponding wave. Lesser frequency results in the fewer number of cycles completed in a given amount of time or to complete one cycle the wave or particle requires more time.
The relation can be derived by using De Broglie’s equation and substituting the values or relations of frequency, wavelength, and speed of light respectively.
Problems based on the relationship between frequency and energy
1. A photon has a frequency of 1015 Hz. Calculate the energy of this photon.
Solution: We can use the energy-frequency relationship for photons: E = h * ν. Given that the frequency (ν) is 1015 Hz and the Planck constant (h) is approximately 6.626 x 10-34 joule-seconds, we can calculate the energy (E):
E = (6.626 x 10-34 J·s) * (1015 Hz)
E = 6.626 x 10-19 J
Therefore, the energy of the photon is approximately 6.626 x 10-19 joules.
2. An electron has a de Broglie wavelength of 2 nm. Calculate the energy associated with this electron.
Solution: We can use the de Broglie wavelength equation, which relates the wavelength (λ) to the momentum (p) of a particle: λ = h / p. Rearranging the equation, we get p = h / λ. The energy (E) of a particle is related to its momentum by E = p2 / (2m), where m is the mass of the particle.
Given that the de Broglie wavelength (λ) is 2 nm (1 nm = 1 x 10-9 m) and the Planck constant (h) is approximately 6.626 x 10-34 J·s, we can calculate the momentum (p):
p = (6.626 x 10-34J·s) / (2 x 10-9 m)
p = 13.252 x 10-25 kg·m/s
Now, we can calculate the energy (E):
E = (13.252 x 10-25 kg·m/s)2 / (2 * mass of electron)
E = 8.738 x 10-51 J
Therefore, the energy associated with the electron is approximately 8.738 x 10-51 joules.
3. A red laser emits light with a wavelength of 650 nm. Calculate the frequency and energy of each photon of this light.
Solution: First, we can calculate the frequency (ν) using the speed of light (c) and the wavelength (λ) through the equation c = λν. Given that the speed of light is approximately 3 x 108 m/s:
ν = c / λ
ν = (3 x 108 m/s) / (650 x 10-9 m)
ν ≈ 4.62 x 1014 Hz
Next, we can use the energy-frequency relationship to calculate the energy (E) of each photon:
E = hν
E = (6.626 x 10-34 J·s) * (4.62 x 1014 Hz)
E ≈ 3.06 x 10-19 J
Therefore, each photon of the red laser light has a frequency of approximately 4.62 x 1014 Hz and an energy of approximately 3.06 x 10-19 joules.
4. An electron moves with a velocity of 2 x 106 m/s. Calculate the de Broglie wavelength and the associated energy of this electron.
Solution: The de Broglie wavelength (λ) of a particle is given by the equation λ = h / p, where h is the Planck constant and p is the momentum of the particle.
First, we calculate the momentum (p) of the electron using its mass (m) and velocity (v):
p = m * v
p = (9.1 x 10-31) * (2 x 106 m/s)
p = 18.2 x 10-25
Next, we can use the de Broglie wavelength equation to calculate the wavelength:
λ = h / p
λ = (6.626 x 10-34) / (18.2 x 10-25)
λ = 0.36 x 10-9
Finally, we can use the energy-momentum relationship for particles to calculate the energy (E) associated with the electron:
E = p2 / (2m)
E = (18.2 x 10-25 )2 / (2 * 9.1 x 10-31)
E = 18.2 x 10-19 J
Q&A
1. What is the relationship between frequency and energy equation?
Ans. The relationship between frequency and energy is
E = h*v
where E is energy, h is Planck’s constant, and v is frequency.
2. Is energy and frequency directly proportional?
Ans. Yes, according to the mathematical expression frequency and energy are directly proportional to each other.
3. What is the relationship between the frequency and energy of the EM wave?
Ans. For an electromagnetic wave, the relation between frequency and energy is
E = h*v
where E is energy, h is Planck’s constant, and v is frequency.
4. Relationship between wavelength frequency and energy.
Ans. The relationship between wavelength, frequency, and energy are :
v = c / λ
E = h*v
E = h* (c/λ)
Where E is energy, v is frequency, h is Planck’s constant, c is the speed of light, and λ is the wavelength.
Summary
Where we denote Frequency as f and time period as T.
Where E is the energy of the particle or photon, h is Planck’s constant, ν is the frequency |
