Know in one minute about Schrodinger Wave EquationErwin Schrodinger an Austrian physicist give Schrodinger Wave Equation in the year 1925. It helped to study the probability of finding a particle at a particular position and with a particular momentum. The Schrodinger wave equation has the following practical applications : ● Describing the behavior of atoms and molecules. ● Predicting the properties of a particle in an external potential field. ● Designing algorithms in quantum computing. ● Making digital models of a quantum system. The formula is : iħ ∂ψ/∂t = Hψ where i is the imaginary unit, ħ is the reduced Planck constant, t is time, ψ is the wave function, and H is the Hamiltonian operator, which is given by: H = -ħ^2/2m ∇^2 + V(x) There also exist some popular variations of the Schrodinger wave equation such as:
|
Introduction
The Schrödinger equation is used to calculate the probability of finding a particle in a particular location at a particular time. The wave function describes the probability amplitude of finding the particle in different locations, and the square of the absolute value of the wave function gives the probability density of finding the particle in a particular location.
In the quantum mechanic when one talks about the position, velocity, and acceleration of any particle, we have certain ways in the present time to calculate its probability or a value that is close to an estimation of the actual value. But the condition was not the same before 1925.
It was the time when scientists were working hard on atomic models, and building up the foundation of Quantum mechanics. Many scientists proposed their studies on the position, acceleration, and velocity of a particle.
Louis de Broglie, the name famous scientist from France, proposed a way to predict the position of a particle in his doctoral thesis in the year 1924. He said that all matter possesses both, the properties of a wave as well as that of a particle. According to him the motion of a particle can be described by a wave equation.
Later, in the year 1925, Erwin Schrodinger an Austrian Physicist derived this equation into a partial differential equation that describes the wave function of a quantum system. The wave function is a mathematical function that represents the probability amplitude of the position and momentum of a particle in a quantum system. In other words, it describes the probability of finding a particle at a particular position and with a particular momentum.
The Schrödinger equation is a non-relativistic equation, meaning that it does not take into account the effects of special relativity. For particles traveling at speeds close to the speed of light, a different equation, known as the Dirac equation, is used.
Derivation and Formula
Let’s now have a look at the formula of the Schrodinger wave equation.
iħ ∂ψ/∂t = Hψ
where i is the imaginary unit, ħ is the reduced Planck constant, t is time, ψ is the wave function, and H is the Hamiltonian operator, which is given by:
H = -ħ^2/2m ∇^2 + V(x)
The also has some other variations, as it can also be written in the following way-
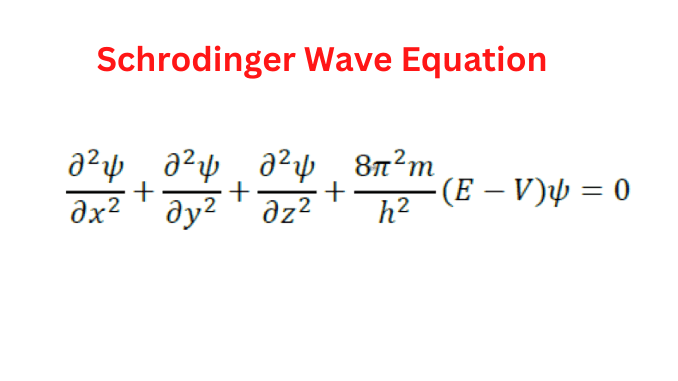
∂2Ψ/∂x2 + ∂2Ψ/∂y2 + ∂2Ψ/∂z2 + 8π2 m(E−V)Ψ/h2=0.

Where,
x,y,z = space co-ordinates
m = mass of the electron
h = Plancks constant
E = Total energy
V = Potential energy
Ψ = wave function of a particle
The Schrödinger equation is a partial differential equation, meaning that it involves derivatives with respect to both time and position. It is a linear equation, which means that if ψ1 and ψ2 are both solutions to the equation, then any linear combination of ψ1 and ψ2 is also a solution.
The wave function ψ in the Schrödinger equation can be used to calculate various properties of the quantum system, such as the expected value of observables like position, momentum, and energy. These calculations are performed using operators that act on the wave function.
Derivation
Energy = Kinetic energy + Potential Energy
E = ½ mv2 + V ——— 1
Now, energy can also be written as :
½ mv2 = ½ (mv)2 / m ——— 2
Putting this value in de Broglies equation –
mv = h/λ
Therefore,
½ mv2 = ½ (h/λ)2 / m
½ mv2 = ½ h2/(λ2 m) ——— 3
Substituting values from equation 3 into equation 1.
E = ½ h2/(λ2 m) + V
E – V = ½ h2/(λ2 m) ——— 4
λ2 = ½ h2/(E – V) m ——— 5
For an electron that moves with wave’s speed, the equation for its wave motion in X direction can be expressed as :
∂2Ψ/∂x2 + 4π2 Ψ/ λ2=0 ——— 6
Substitute the values from Equation 5 into Equation 6
Therefore, we get:
∂2Ψ/∂x2 + 8π2 m(E−V)Ψ/h2 = 0 ——— 7
Hence, for all three axes i.e. x,y,z we can write the equation as:
∂2Ψ/∂x2 + ∂2Ψ/∂y2 + ∂2Ψ/∂z2 + 8π2 m(E−V)Ψ/h2=0.

Uses or Application
Schrodinger wave equation plays a very crucial role in the field of quantum mechanics and the technologies which are being developed using it. Let us look at some of its applications that are being practiced in the modern world.
1. Describing the behavior of atoms and molecules
One can find out the behavior i.e. its velocity, position, acceleration, momentum, etc. of a particle such as an electron using the Schrodinger wave equation. With the help of such information, we can study the physical and chemical properties of a material.
2. Predicting the behavior of particles in potential fields
In order to study a matter or particle’s behavior under the influence of some potential field or even magnetic fields, we use Schrodingers wave equation. By solving it for a given potential, we can determine the allowed energy levels and corresponding wave functions for the particle, which can be used to calculate the behavior of the particle. This becomes important when we want to induce a material into the construction of some kind of mechanism in which potential fields can be present.
3. Simulating quantum systems
This equation can be used to form a digital model of quantum mechanics on the computer by using numerical methods such as the finite element method or the finite difference method. These simulations can be used to study the behavior of complex systems that cannot be solved analytically.
4. Designing quantum algorithms
The equation is used to design quantum algorithms, which are used in quantum computing. By understanding the behavior of quantum systems, we can design algorithms that take advantage of quantum phenomena such as superposition and entanglement, and solve problems that would be intractable using classical computers.
Some Sample Problems
1. What does ‘H’ stands for, in the Schrodinger wave equation?
In the Schrodinger wave equation ‘H’ stands for the Hamiltonian operator.
Hamiltonian operator is a mathematical operator used in quantum mechanics to represent the total energy of a quantum system. It is denoted by the symbol H and is defined as the sum of the kinetic and potential energy of the system.
2. On what principles is the Schrodinger wave equation based?
The Schrodinger wave equation is based on the de Broglie wave equation. Erwin Schrodinger then derived this equation to give the wave equation which helps us to study the behavior of a particle, predict its position, momentum, etc.
3. What are the practical applications of the Schrodinger wave equation?
The Schrodinger wave equation has the following practical applications :
- Describing the behavior of atoms and molecules.
- Predicting the properties of a particle in an external potential field.
- Designing algorithms in quantum computing.
- Making digital models of a quantum system.
4. Give some limitations of the Schrodinger wave equation.
The following are some limitations of the wave equation :
- The Schrodinger wave equation does not take special relativity into account, due to which it becomes non-relativistic.
- The equation cannot describe all interactions.
- It cannot describe entanglement, i.e. where two particles become correlated in such a way that it cannot be explained using classical physics.
Q&A
1. What is Schrodinger wave equation?
The Schrodinger wave equation is a mathematical expression that describes the wave function of a quantum system in the form of the partial differential equation. It describes the probability of finding a particle at a particular position and with particular momentum.
2. When was the Schrodinger equation created?
The Schrodinger wave equation was derived by Erwin Schrodinger in the year 1925.
3. How was Schrodinger equation derived?
Schrodinger wave equation was derived using de Broglie’s wave equation.
4. How to derive Schrodinger wave equation?
In order to derive the Schrodinger wave equation one should follow these steps:
- Calculate the total energy for the particle.
- Substitute the values for kinetic energy in the equation.
- Use the de broglie wave equation to find kinetic energy.
- Substitute the values and find the equation of wave motion for a particle.
- Write the equation for wave motion with respect to all three axes.
For more clarity check out the derivation mentioned above.
5. Who is Schrodinger and what did he do?
Erwin Schrodinger was an Austrian physicist. He worked in the field of quantum systems, and his work and researches were very important contributions to the field. The wave equation derived by him was among his valuable contributions.
Written By: Bharat Awasthi
